Nachweis von Ankern gemäß AISC

Die Kräfte in Ankern werden durch Finite-Elemente-Analyse ermittelt, aber die Tragfähigkeiten werden nach den Normanforderungen von ACI 318-14 - Kapitel 17 überprüft.

Es ist nur LFRD verfügbar. Ankerstäbe sind gemäß AISC 360-16 – J9 und ACI 318-14 – Kapitel 17 konstruiert. Folgende Tragfähigkeiten von Ankerschrauben werden bewertet:

• Steel strength of anchor in tension ϕN_sa,
• Concrete breakout strength in tension ϕN_cbg,
• Concrete pullout strength ϕN_p,
• Concrete side-face blowout strength ϕN_sb,
• Steel strength of anchor in shear ϕV_sa,
• Concrete breakout strength in shear ϕV_cbg,
• Concrete pryout strength of anchor in shear ϕV_cp.

Der Nutzer muss den Betonzustand (gerissen oder ungerissen – ohne Risse im Betriebszustand) und die Art der Anker (mit oder ohne Unterlegscheiben) wählen.

Stahlfestigkeit eines Zugankers

Die Stahlfestigkeit eines Zugankers wird gemäß ACI 318-14 – 17.4.1 ermittelt als

ϕN_sa = ϕ A_se,N f_uta

Wo:

• ϕ = 0,7 – strength reduction factor for anchors in tension according to ACI 318-14 – 17.3.3, änderbar in den Normeinstellungen
• A_se,N – tensile stress area
• f_uta – specified tensile strength of anchor steel and shall not be greater than 1,9 f_ya and 125 ksi

Betonausbruchfestigkeit

Die Betonausbruchfestigkeit wird gemäß dem Concrete Capacity Design (CCD) in ACI 318-14 – Kapitel 17 bemessen. Bei der CCD-Methode wird der Betonkegel als in einem Winkel von ungefähr 34° gebildet betrachtet (1 vertikale bis 1,5 horizontale Neigung). Zur Vereinfachung wird der Kegel im Grundriss eher quadratisch als rund angesehen. Es wird angenommen, dass die Betonausbruchspannung beim CCD-Verfahren mit zunehmender Größe der Ausbruchfläche abnimmt. Folglich ist die Festigkeitszunahme des Ausbruchs beim CCD-Verfahren proportional zur Einbettungstiefe hoch 1,5. Anker, deren Betonkegel sich überlappen, bilden eine Gruppe von Ankern, die einen gemeinsamen Betonkegel bilden. Beachten Sie, dass für die Kapazitätsbemessung von Beton keine äquivalente ASD-Lösung existiert.

\[ \phi N_{cbg} = \phi \frac{A_{Nc}}{A_{Nco}} \psi_{ec,N} \psi_{ed,N} \psi_{c,N} \psi_{cp,N} N_b \]

Wo:

• ϕ = 0,7 – strength reduction factor for anchors in tension according to ACI 318-14 – 17.3.3, änderbar in den Normeinstellungen
• A_Nc – actual concrete breakout cone area for a group of anchors that create common concrete cone
• A_Nco = 9 h_ef² – concrete breakout cone area for single anchor not influenced by edges
• \( \psi_{ec,N} = \frac{1}{1+\frac{2 e'_N}{3 h_{ef}}} \) – modification factor for anchor groups loaded eccentrically in tension; in the case where eccentric loading exists about two axes, the modification factor Ψ_ec,N is calculated for each axis individually and the product of these factors is used
• \( \psi_{ed,N} = \min \left ( 0,7 + \frac{0,3 c_{a,min}}{1,5 h_{ef}}, 1 \right ) \) – modification factor for edge distance
• c_a,min – smallest distance from the anchor to the edge
• Ψ_c,N – modification factor for concrete conditions; Ψ_c,N =1 for cracked concrete, Ψ_c,N =1,25 for non-cracked concrete
• Ψ_cp,N = min (c_a,min / c_ac,1) – modification factor for splitting for post-installed anchors designed for uncracked concrete without supplementary reinforcement to control splitting; Ψ_cp,N = 1 for all other cases
• \( N_b = k_c \lambda_a \sqrt{f'_c} h_{ef}^{1.5} \) – basic concrete breakout strength of a single anchor in tension in cracked concrete; for cast-in anchors and 11 in. ≤ h_ef ≤ 25 in. \( N_b = 16 \lambda_a \sqrt{f'_c} h_{ef}^{5/3} \)
• k_c = 24 for cast-in anchors
• h_ef – depth of embedment; according to Chapter 17.4.2.3 in ACI 318-14, the effective embedment depth h_ef is reduced to \( h_{ef} = \max \left ( \frac{c_{a,max}}{1.5}, \frac{s}{3} \right ) \) if anchors are located less than 1,5 h_ef from three or more edges
• s – spacing between anchors
• c_a,max – maximum distance from an anchor to one of the three close edges
• λ_a = 1 – modification factor for lightweight concrete
• f'_c – concrete compressive strength [psi]

Gemäß ACI 318-14 – 17.4.2.8 wird bei Kopfankern die projizierte Oberfläche A_Nc aus dem wirksamen Umfang der Unterlegscheibe ermittelt, als kleineren Wert von d_a + 2 t_wp oder d_wp, wobei:

• d_a – anchor diameter
• d_wp – washer plate diameter or edge size
• t_wp – washer plate thickness

Die Ankergruppe wird gegen die Summe der Zugkräfte in Ankern geprüft, die auf Zug belastet sind und einen gemeinsamen Betonkegel bilden.

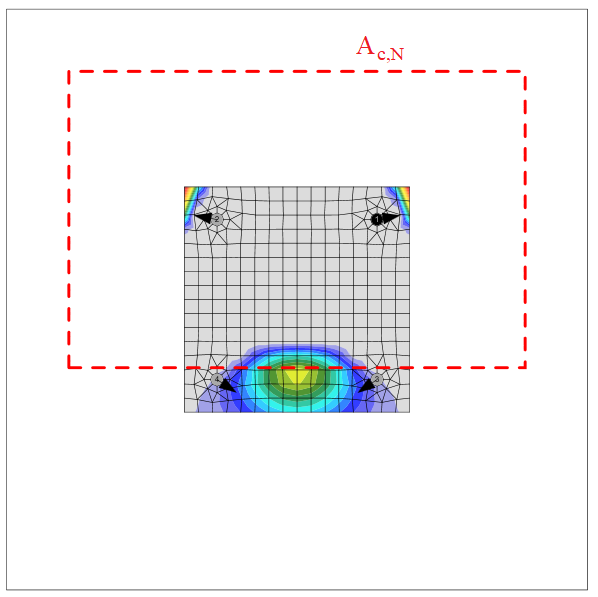
Der Bereich des Betonausbruchskegels für eine Gruppe von durch Zug belasteten Ankern, die einen gemeinsamen Betonkegel A_c,N bilden, ist durch eine rote gestrichelte Linie dargestellt.

Nach ACI 318-14 – 17.4.2.9, wenn die Ankerbewehrung nach ACI 318-14 – 25 auf beiden Seiten der Ausbruchfläche ausgeführt wird, wird angenommen, dass die Ankerbewehrung die Zugkräfte überträgt und die Betonbruchfestigkeit wird nicht ausgewertet.

Betonauszugsfestigkeit

Die Betonauszugsfestigkeit eines Ankers ist in ACI 318-14 – 17.4.3 definiert als

ϕN_pn = ϕΨ_c,P N_p

Wo:

• ϕ = 0,7 – strength reduction factor for anchors in tension gemäß ACI 318-14 – 17.3.3, änderbar in den Normeinstellungen
• Ψ_c,P – modification factor for concrete condition; Ψ_c,P = 1,0 for cracked concrete, Ψ_c,P = 1,4 for non-cracked concrete
• N_P = 8 A_brg f'_c for headed anchor
• A_brg – bearing area of the head of stud or anchor bolt
• f'_c – concrete compressive strength

Die Betonauszugsfestigkeit für andere Ankertypen als Kopfanker wird in der Software nicht bewertet und muss vom Hersteller angegeben werden.

Betonseitenausbruchfestigkeit

Die Betonausbruchfestigkeit eines Kopfankers unter Zug ist in ACI 318-14 – 17.4.4 definiert als

\[ \phi N_{sb} = \phi 160 c_{a1} \sqrt{A_{brg}} \sqrt{f'_c} \]

The concrete side-face blowout strength is multiplied by one of reduction factors:

• \( \frac{1+\frac{c_{a2}}{c_{a1}}}{4} \le 1 \)
• \( \frac{1+\frac{s}{6 c_{a1}}}{2} \le 1 \)

Wo:

• ϕ = 0,7 – strength reduction factor for anchors in tension gemäß ACI 318-14 – 17.3.3, änderbar in den Normeinstellungen
• c_a1 – shorter distance from the centreline of an anchor to an edge
• c_a2 – longer distance, perpendicular to c_a1, from the centreline of an anchor to an edge
• A_brg – bearing area of the head of stud or anchor bolt
• f'_c – concrete compressive strength
• h_ef – depth of embedment; according to Chapter 17.4.2.3 in ACI 318-14, the effective embedment depth h_ef is reduced to \( h_{ef} = \max \left ( \frac{c_{a,max}}{1.5}, \frac{s}{3} \right ) \) if anchors are located less than 1.5 h_ef from three or more edges
• s – spacing between two adjacent anchors near one edge

Stahlfestigkeit bei Scherung

Die Stahlscherfestigkeit wird nach ACI 318-14 – 17.5.1 ermittelt als

ϕV_sa = ϕ 0,6 A_se,V f_uta

Wo:

• ϕ = 0,65 – strength reduction factor for anchors in tension gemäß ACI 318-14 – 17.3.3, änderbar in den Normeinstellungen
• A_se,V – tensile stress area
• f_uta – specified tensile strength of anchor steel and shall not be greater than 1,9 f_ya and 125 ksi

If mortar joint is selected, steel strength in shear V_sa is multiplied by 0.8 (ACI 318-14 – 17.5.1.3).

Die Scherkraft am Hebelarm, die bei Fußplatten mit übergroßen Löchern und Unterlegscheiben oder auf der Oberseite der Fußplatte angebrachten Platten zur Übertragung der Scherkraft vorhanden ist, wird nicht berücksichtigt.

Betonausbruchfestigkeit bei Scherankern

Die Betonausbruchfestigkeit eines Ankers oder einer Ankergruppe auf Scherung wird gemäß ACI 318 14 – 17.5.2 bemessen.

\[ \phi V_{cbg} = \phi \frac{A_V}{A_{Vo}} \psi_{ec,V} \psi_{ed,V} \psi_{c,V} \psi_{h,V} \psi_{\alpha,V} V_b \]

Wo:

• ϕ = 0.65 – strength reduction factor for anchors in shear gemäß ACI 318-14 – 17.3.3, änderbar in den Normeinstellungen
• A_v – projected concrete failure area of an anchor or group of anchors
• A_vo – projected concrete failure area of one anchor when not limited by corner influences, spacing or member thickness
• \( \psi_{ec,V} = \frac{1}{1+\frac{2 e'_V}{3 c_{a1}}} \) – modification factor for anchor groups loaded eccentrically in shear
• \( \psi_{ed,V} = 0,7 + 0,3 \frac{c_{a2}}{1.5 c_{a1}} \le 1,0 \) – modification factor for edge effect
• Ψ_c,V – modification factor for concrete condition; Ψ_c,V = 1,0 for cracked concrete, Ψ_c,V = 1,4 for non-cracked concrete
• \( \psi_{h,V} = \sqrt{\frac{1,5 c_{a1}}{h_a}} \ge 1 \) – modification factor for anchors located in a concrete member where h_a < 1,5 c_a1
• \( \psi_{\alpha ,V} = \sqrt{\frac{1}{(\cos \alpha_V )^2 + (0,5 \sin \alpha_V)^2}} \) – modification factor for anchors loaded at an angle 90° − α_V with the concrete edge; in ACI 318-14 – 17.5.2.1 are only discrete values, equation is taken from FIB bulletin 58 – Design of anchorages in concrete (2011)
• h_a – height of a failure surface on the concrete side
• \( V_b = \min \left ( 7 \left ( \frac{l_e}{d_a} \right )^{0,2} \lambda_a \sqrt{d_a} \sqrt{f'_c} c_{a1}^{1,5}, 9 \lambda_a \sqrt{d_a} \sqrt{f'_c} c_{a1}^{1,5} \right ) \)
• l_e = h_ef ≤ 8 d_a – load-bearing length of the anchor in shear
• d_a – anchor diameter
• f'_c – concrete compressive strength
• c_a1 – edge distance in the direction of load; according to Cl. 17.5.2.4, for a narrow member, c_2,max < 1,5 c₁ that is also deemed to be thin, h_a < 1,5 c₁, c'₁ is used in previous equations instead of c₁; the reduced c'₁ = max (c_2,max / 1,5, h_a / 1,5, s_c,max / 3)
• c_a2 – edge distance in the direction perpendicular to load
• c_2,max – largest edge distance in the direction perpendicular to load
• s_c,max – maximum spacing perpendicular to direction of shear, between anchors within a group

If c_a2 ≤ 1,5 c_a1 and h_a ≤ 1,5 c_a1, \( c_{a1}= \max \left ( \frac{c_{a2}}{1,5}, \frac{h_a}{1,5}, \frac{s}{3} \right ) \), where s is the maximum spacing perpendicular to direction of shear, between anchors within a group.

Gemäß ACI 318-14 – 17-5.2.9, wenn die Ankerbewehrung nach ACI 318-14 – 25 auf beiden Seiten der Ausbruchsfläche auftritt, wird angenommen, dass die Ankerbewehrung die Scherkräfte überträgt und die Betonausbruchfestigkeit wird nicht ausgewertet.

Betonauszugfestigkeit bei Scherankern

Die Betonausbruchfestigkeit wird gemäß ACI 318-14 – 17.5.3 bemessen.

ϕV_cp = ϕk_cp N_cp

Wo:

• ϕ = 0,65 – strength reduction factor for anchors in shear gemäß ACI 318-14 – 17.3.3, änderbar in den Normeinstellungen
• k_cp = 1,0 for h^ef < 2,5 in., k_cp = 2,0 for h_ef ≥ 2,5 in
• N_cp = N_cb (concrete breakout strength – all anchors are assumed in tension) in case of cast-in anchors

Nach ACI 318-14 – 17.4.2.9, wenn die Ankerbewehrung nach ACI 318-14 – 25 auf beiden Seiten der Ausbruchsfläche auftritt, wird angenommen, dass die Ankerbewehrung die Zugkräfte überträgt und die Betonbruchfestigkeit wird nicht ausgewertet.

Interaktion von Zug- und Scherkräften

Die Interaktion von Zug- und Scherkräften wird gemäß ACI 318-14 – R17.6 bewertet.

\[ \left ( \frac{N_{ua}}{N_n} \right )^{\zeta} + \left ( \frac{V_{ua}}{V_n} \right )^{\zeta} \le 1,0 \]

Wo:

• N_ua and V_ua – design forces acting on an anchor
• N_n and V_n – the lowest design strengths determined from all appropriate failure modes
• ς = 5 / 3

Abstandsanker

Das Stabelement ist nach AISC 360-16 ausgelegt. Die Interaktion der Scherkraft wird vernachlässigt, da die Mindestlänge des Ankers, um die Mutter unter die Fußplatte zu montieren, gewährleistet, dass der Anker beim Biegen versagt, bevor die Scherkraft die Hälfte der Schertragfähigkeit erreicht und die Interaktion der Scherkraft vernachlässigbar ist (bis zu 7%). Die Interaktion von Biegemoment und Druck- bzw. Zugkraft wird konservativ als linear angenommen. Auswirkungen zweiter Ordnung werden nicht berücksichtigt.

Schertragfähigkeit (AISC 360-16 – G):

\( V_n = \frac{0,6 A_V F_y}{\Omega_V} \)    (ASD)

\( V_n = \phi_V 0,6 A_V F_y \)    (LRFD)

• A_V = 0,844 ∙ A_s – the shear area
• A_s – bolt area reduced by threads
• F_y – bolt yield strength
• Ω_V – safety factor, recommended value is 2
• ϕ_V – resistance factor, recommended value is 0,75

Zugtragfähigkeit (AISC 360-16 – D2):

\( P_n = \frac{A_s F_y}{\Omega_t} \)    (ASD)

\( P_n = \phi_t A_s F_y \)    (LRFD)

• Ω_t – safety factor, recommended value is 2
• ϕ_t – resistance factor, recommended value is 0,75

Drucktragfähigkeit (AISC 360-16 – E3)

\( P_n = \frac{F_{cr} A_s}{\Omega_c} \)    (ASD)

\( P_n = \phi_c F_{cr} A_s \)    (LRFD)

• \( F_{cr} = 0.658^{\frac{F_y}{F_e}} F_y \) for \( \frac{L_c}{r} \le 4.74 \sqrt{\frac{E}{F_y}} \),  \( F_{cr} = 0.877 F_e \) for \( \frac{L_c}{r} > 4.74 \sqrt{\frac{E}{F_y}} \) – critical stress
• \( F_e = \frac{\pi^2 E} {\left ( \frac{L_c}{r} \right) ^2} \) – elastic buckling stress
• L_c = 2 ∙ l – buckling length
• l – length of the bolt element equal to half the base plate thickness + gap + half the bolt diameter
• \( r= \sqrt{\frac{I}{A_s}} \) – radius of gyration of the anchor bolt
• \( I= \frac{\pi d_s^4}{64} \) – moment of inertia of the bolt
• Ω_c – safety factor, recommended value is 2
• ϕ_c – resistance factor, recommended value is 0,75

Biegetragfähigkeit (AISC 360-16 – F11):

\( M_n = \frac{Z F_y}{\Omega_b} \le \frac{1.6 S_x F_y}{\Omega_b} \)   (ASD)

\( M_n = \phi_b Z F_y \le 1.6 \phi_b S_x F_y \)   (ASD)

• \( Z = \frac{d_s^3}{6} \) – plastic section modulus of the bolt
• \( S_x= \frac{2 I}{d_s} \) – elastic section modulus of the bolt
• Ω_c – safety factor, recommended value is 2
• ϕ_c – resistance factor, recommended value is 0,75

Lineare Interaktion:

\[ \frac{N}{P_n}+\frac{M}{M_n} \le 1 \]

• N – tensile (positive) or compressive (negative sign) factored force
• P_n – tensile (positive) or compressive (negative sign) design or allowable strength
• M – factored bending moment
• M_n – the design or allowable bending resistance