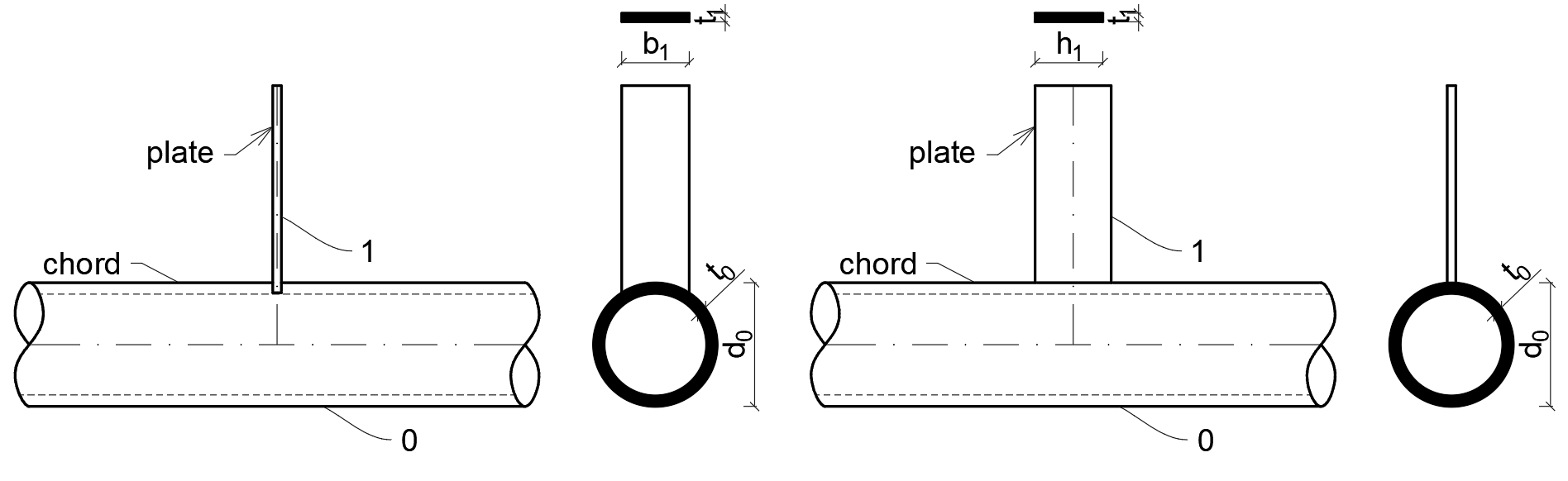
Plate to circular hollow section
Failure mode method
Uniplanar welded plate to circular hollow sections T-joints predicted by CBFEM are verified to FMM in this chapter. In CBFEM, the design resistance is limited by reaching 5 % of strain or a force corresponding to 3 % d0 joint deformation, where d0 is chord diameter. The FMM is based on the peak load limit or 3 % d0 deformation limit; see Lu et al. (1994). The welds, designed according to EN 1993‑1‑8:2006, are not the weakest components in the joint.
Chord plastification
The design resistance of a CHS chord face is determined using the method given by FMM model in Ch. 9 of prEN 1993-1-8:2020 and in ISO/FDIS 14346; see Fig. 7.3.1. The design resistance of the axially loaded welded plate to CHS joint is:
T joint
Transverse
\[ N_{1,Rd} = 2.5 C_f f_{y0} t_0^2 (1+3 \beta^2) \gamma^{0.35} Q_f / \gamma_{M5} \]
Longitudinal
\[ N_{1,Rd} = 7.4 C_f f_{y0} t_0^2 (1+0.4 \eta) Q_f / \gamma_{M5} \]
X joint
Transverse
\[ N_{1,Rd} = 2.1 C_f f_{y0} t_0^2 (1+3 \beta^2) \gamma^{0.25} Q_f / \gamma_{M5} \]
Longitudinal
\[ N_{1,Rd} = 3.5 C_f f_{y0} t_0^2 (1+0.4 \eta^2) \gamma^{0.1} Q_f / \gamma_{M5} \]
where:
- fy,i – yield strength of member i (i = 0,1,2 or 3)
- ti – thickness of the wall of CHS member i (i = 0,1,2 or 3)
- \(\beta\) – ratio of the mean diameter or width of brace members, to that of the chord
- \(\eta\) – ratio of the brace member depth to the chord diameter or width
- \(\gamma\) – ratio of a chord width or diameter to twice its wall thickness
- Qf – chord stress factor
- Cf – material factor
- \(\gamma_{M5}\) – partial factor for resistance of joints in hollow section lattice girders
- Ni,Rd – design resistance of a joint expressed in terms of the internal axial force in member i (i = 0,1,2 or 3)
Fig. 7.3.1 Examined failure mode - chord plastification
Range of validity
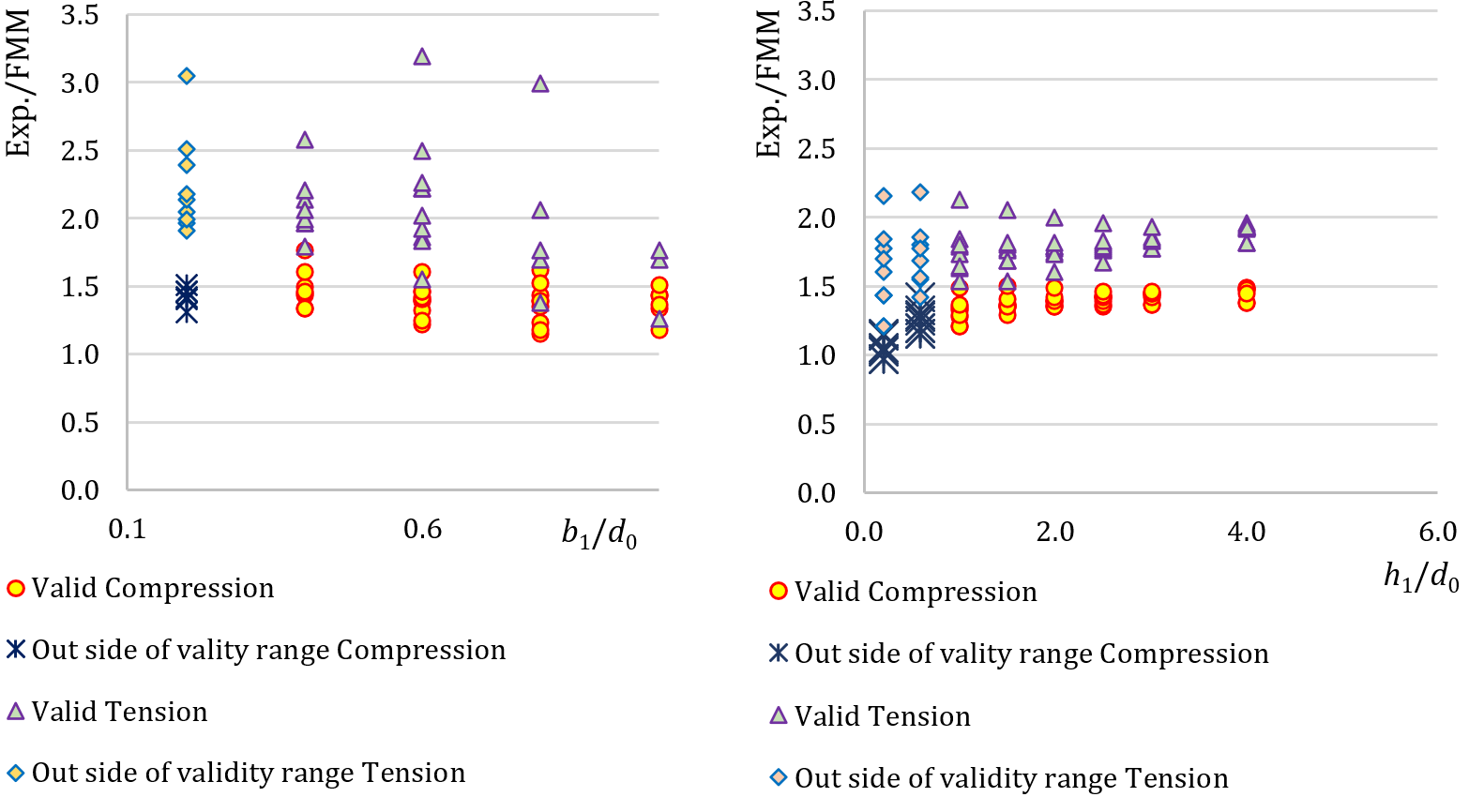
CBFEM was verified for typical joints of the welded circular hollow sections. The range of validity for these joints is defined in Table 7.8 of prEN 1993-1-8:2020; see Tab 7.3.1. The same range of validity is applied to CBFEM model. Outside the range of validity of FMM, an experiment should be prepared for validation or verification performed for verification according to a validated research model.
Tab. 7.3.1 Range of validity for method of failure modes
| General | \(0.2 \le \frac{d_i}{d_0} \le 1.0 \) | \( \theta_i \ge 30^{\circ} \) | \(-0.55 \le \frac{e}{d_0} \le 0.25 \) |
| \(g \ge t_1+t_2 \) | \(f_{yi} \le f_{y0} \) | \( t_i \le t_0 \) |
| Chord | Compression | Class 1 or 2 and \(10 \le d_0 / t_0 \le 50 \) (but for X joints: \( d_0/t_0 \le 40 \)) |
| Tension | \(10 \le d_0 / t_0 \le 50 \) (but for X joints: \( d_0/t_0 \le 40 \)) | |
| Transverse plate | \(0.25\le\beta=b_1/d_0\le1\) | |
| Longitudinal plate | \(0.6\le\eta=h_1/d_0\le4 \) |
Validation
In this chapter, the CBFEM is validated to the FMM models of plate to CHS T-joints described in prEN 1993-1-8:2020. The models are compared against the data from mechanical tests in Tabs 7.3.2–7.3.3 with resistance based on deformation limit. Material and geometric properties of numerical tests are described in (Voth A.P. and Packer A.J., 2010). The experiments out of range of validity are marked in tables by star * and in graph indicated to show the quality of the boundary conditions.
Tab. 7.3.2 Geometric properties, material properties, and resistances of connections from experiments and FMM models for transverse T-joint
| ID | Reference | d0 [mm] | t0 [mm] | h1 [mm] | h1/d0 [-] | d0/t0 [-] | fy0 [MPa] |
| TPT 1 | Washio et al. (1970) | 165,2 | 5,2 | 115,6 | 0,7 | 31,8 | 308,0 |
| TPT 2 | Washio et al. (1970) | 165,2 | 5,2 | 148,7 | 0,9 | 31,8 | 308,0 |
| TPT 3 | Washio et al. (1970) | 139,8 | 3,5 | 125,8 | 0,9 | 39,9 | 343,0 |
| TPT 4 | Voth et al. (2012) | 219,2 | 4,5 | 100,3 | 0,5 | 48,8 | 388,8 |
| ID | Nu,exp [kN] | Branch type | Nu,exp/(t02·fy0) | N1,prEN/(t02·fy0) | Nu,exp/N1,prEN |
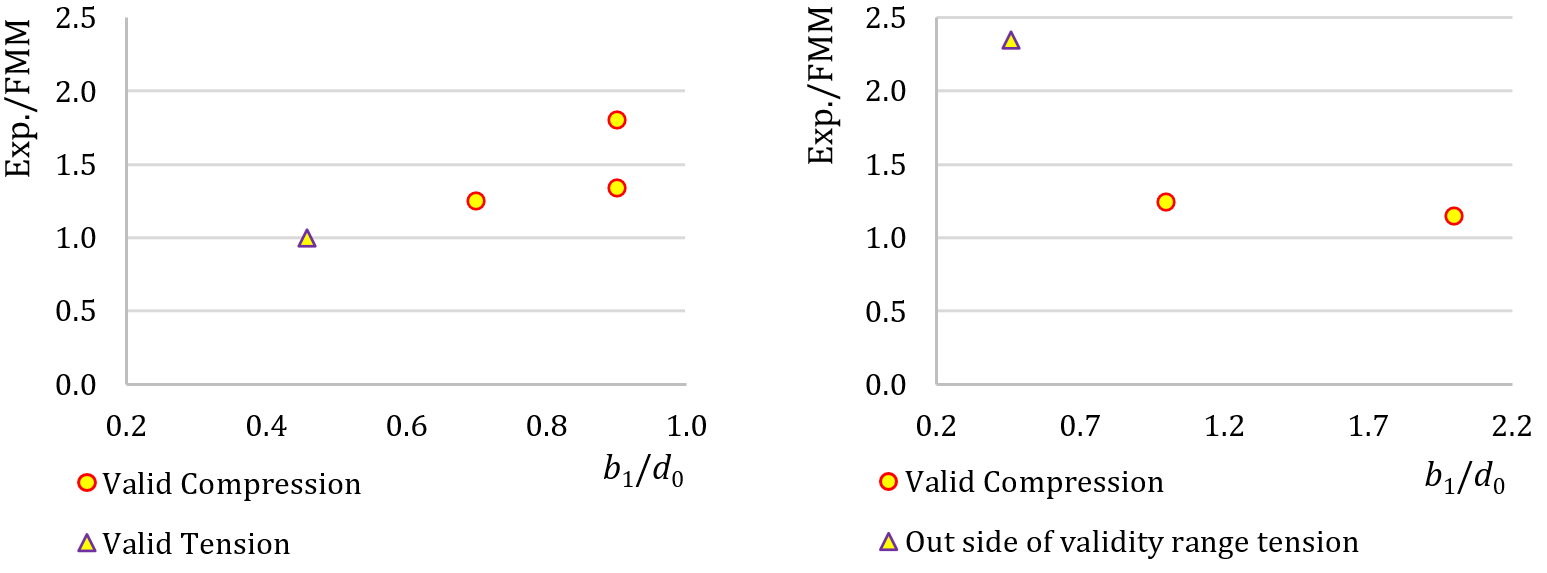
| TPT 1 | 169,4 | Compression | 20,34 | 16,25 | 1,25 |
| TPT 2 | 250,5 | Compression | 30,08 | 22,58 | 1,33 |
| TPT 3 | 184,8 | Compression | 43,98 | 24,45 | 1,80 |
| TPT 4 | 282,5 | Tension | 36,04 | 12,45 | 2,89 |
Tab. 7.3.3 Geometric properties, material properties, and resistances of connections from experiments and FMM models for longitudinal T-joint
| ID | Reference | d0 [mm] | t0 [mm] | h1 [mm] | h1/d0 [-] | d0/t0 [-] | fy0 [MPa] |
| TPL 1 | Washio et al. (1970) | 165,2 | 5,2 | 165,2 | 1,0 | 31,8 | 308,0 |
| TPL 2 | Washio et al. (1970) | 165,2 | 5,2 | 330,4 | 2,0 | 31,8 | 308,0 |
| *TPL 3 | Voth et al. (2012) | 219,2 | 4,5 | 99,9 | 0,5 | 48,8 | 388,8 |
| ID | Nu,exp [kN] | Branch type | Nu,exp/(t02·fy0) | N1,prEN/(t02·fy0) | Nu,exp/N1,prEN |
| TPL 1 | 107,6 | Compression | 12,92 | 10,36 | 1,25 |
| TPL 2 | 127,4 | Compression | 15,30 | 13,32 | 1,15 |
| *TPL 3 | 160,6 | Tension | 20,49 | 8,75 | 2,34 |
Fig. 7.3.2 Validation of FMM to mechanical experiments for transverse T-type plate-to-CHS connections (left) and to longitudinal T-type plate-to-CHS connections (right)
Fig. 7.3.3 Validation of FMM to mechanical experiments for transverse T-type plate-to-CHS connections (left) and longitudinal T-type plate-to-CHS connections (right)
The validation shown in Figs 7.3.2 and 7.3.3 demonstrates that differences to experiments are at least 15 % generally to the safe side. The experiments out of the range of validity are included and marked. The results indicate the good quality of the chosen boundary conditions.
Uniplanar plate T-joint
An overview of the considered examples in the study is given in the Tab. 7.3.4. Selected cases cover a wide range of joint geometric ratios. Geometry of the joints with dimensions is shown in Fig. 7.3.4. Plate thickness is 15 mm in all cases covered in this study.
Tab. 7.3.4 Examples overview
| Example | Chord | Plate orientation | Plate width | Material | ||
| Section | - | b1/h1 | fy | fu | E | |
| [mm] | [MPa] | [MPa] | [GPa] | |||
| 1 | CHS219,1/4,5 | Transverse | 80 | 355 | 490 | 210 |
| 2 | CHS219,1/5,0 | Transverse | 80 | 355 | 490 | 210 |
| 3 | CHS219,1/4,5 | Longitudinal | 180 | 355 | 490 | 210 |
| 4 | CHS219,1/4,5 | Longitudinal | 250 | 355 | 490 | 210 |
| 5 | CHS219,1/4,5 | Longitudinal | 380 | 355 | 490 | 210 |
| 6 | CHS219,1/4,5 | Longitudinal | 500 | 355 | 490 | 210 |
Fig. 7.3.4 Dimensions of plate to CHS T joint, transverse (left) and longitudinal (right)
Verification
The results of resistance and design failure mode of the FMM are compared with the results of CBFEM in Tab. 7.3.5 and in Fig. 7.3.5.
Tab. 7.3.5 Verification of prediction of resistances by CBFEM on FMM
| Example | FMM | Mode of failure | CBFEM | Mode of failure | diff. |
| [kN] | [kN] | [%] | |||
| 1 | 76,9 | Chord plastification | 77,3 | Chord plastification | 0,5 |
| 2 | 91,5 | Chord plastification | 90,6 | Chord plastification | 1,0 |
| 3 | 70,7 | Chord plastification | 65,2 | Chord plastification | 8,4 |
| 4 | 77,5 | Chord plastification | 71,9 | Chord plastification | 7,8 |
| 5 | 90,1 | Chord plastification | 82,8 | Chord plastification | 8,8 |
| 6 | 101,8 | Chord plastification | 93,0 | Chord plastification | 9,4 |
The study shows good agreement for the applied load cases. The results are summarized in a diagram comparing CBFEM’s and FMM’s design resistances; see Fig. 7.3.5. The results show that the difference between the two calculation methods is in all cases less than 7 %.
Fig. 7.3.5 Verification of CBFEM to FMM for the uniplanar Plate to CHS T-joint
Benchmark example
Inputs
Chord
- Steel S355
- Section CHS219.1/4,5
Brace
- Steel S355
- Plate 80/15 mm
- Angle between the brace member and the chord 90° (transversal)
Weld
- Butt weld around the brace
Loaded
- By force to brace in compression
Mesh size
- 64 elements along surface of the circular hollow member
Outputs
- The design resistance in compression is NRd = 77,3 kN
- The design failure mode is chord plastification
Fig. 7.3.6 Boundary conditions for the uniplanar Plate to CHS T-joint