First, let's start with a brief description of our concrete design software. This article is mainly about prestressed concrete design in the Detail application which is generally developed for discontinuity regions design or for the design of members containing discontinuity regions such as openings, dapped ends etc.
For comparison of results, we will use the Beam application whose purpose, as you can guess from the name, is concrete beam design.
Secondly, we need to define a few assumptions and restrictions to understand the design of prestressed concrete beams in Detail better.
- The Time Depended Analysis (TDA) is not implemented in the Detail application. On the other hand, TDA is implemented in the Beam app. for the design of prestressed concrete beams.
- TDA can be simulated in Detail using the creep coefficient and the increments.
- Shrinkage and temperature loads are not implemented in the Detail
- The concrete in tension in the Detail is excluded. So for our comparison, we need to have the beam without cracks. Of course, the same approach can be used generally for beams affected by cracks, but the results will not be then the same in the Beam because only linear calculation is provided in the Beam.
Increments
Before we go through the example, we need to understand how the increments work for prestressed concrete design in the Detail.
There are 3 load types that are applied to the model in three increments in the Detail app.
- Prestressing - for increment P
- Permanent - for increment G
- Variable - for increment V
If you create a combination containing load cases of all load types, the whole portion of Prestressing load type will be applied in the first increment P, the whole portion of Permanent load type will be applied in the second increment G, and the whole portion of Variable load type will be applied in the third increment V.
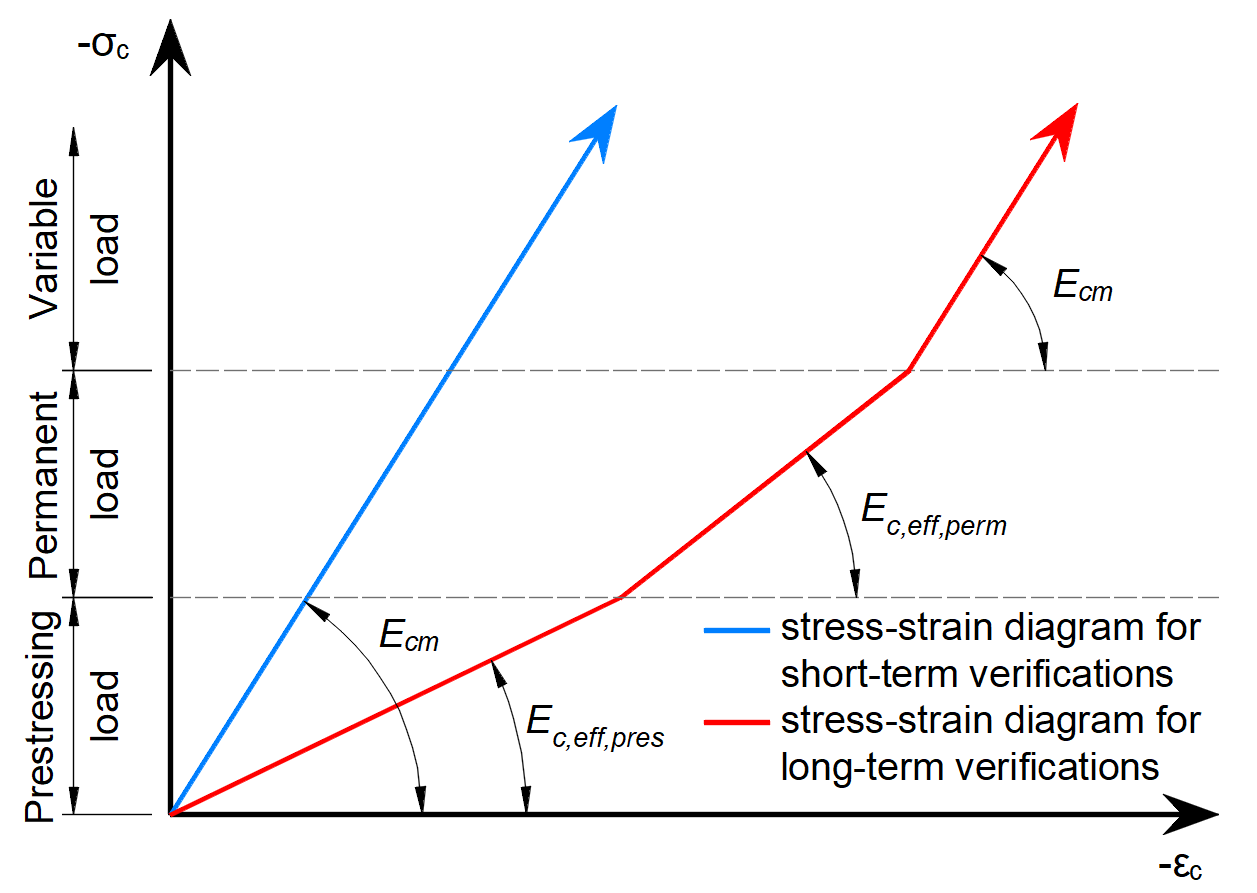
The reason why there are increments is that the different material models (different modules of elasticity) are used for SLS calculations (for ULS there is only one material model defined in Material model (EN)).
As you can see there are three modules of elasticity:
- Ec,eff,press = Ecm / (1+φpress) - Effective modulus of elasticity of concrete for P increment
- Ec,eff,perm = Ecm / (1+φperm) - Effective modulus of elasticity of concrete for G increment
- Ecm - Secant modulus of elasticity of concrete
Where the φpress and φperm are the creep coefficients for the P and G increments. The coefficients can be set in Materials & models.
Please note that for short-term effects only Ecm is used. It is valid for all three increments. And the long-term loss is taken into account only for long-term effects.